 A J 8 4
A J 8 4 K T 6
K T 6 T
T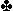 K T 6 4 2
K T 6 4 2 6 3
6 3 A 5 4 3
A 5 4 3 J 5
J 5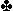 A Q J 8 5
A Q J 8 5On this deal from the Tuesday 23 February Junior Fund game, a thoughtful declarer can win one more trick than one who gives up or gets sloppy.
 A J 8 4 A J 8 4 K T 6 K T 6 T T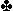 K T 6 4 2 K T 6 4 2 | ||
 6 3 6 3 A 5 4 3 A 5 4 3 J 5 J 5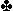 A Q J 8 5 A Q J 8 5 |
At one table, North declared 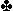
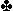
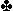


Not quite. It pays to distinguish between absolutely sure losers, and card combinations where there is even a tiny chance of avoiding the loss of a trick. On this deal, there is nothing to be done about the diamond or spade losers but there is a small chance of not losing a heart.
If you look up KTx opposite Axxx in a table of suit combinations, it'll tell you that the best play to win 3 tricks out of 4 in this suit is to lead small toward the king-ten; you will be able to set up your 4th heart if they break 3-3 or if West has QJxx(x).
But on this deal, that's not the way to play the hearts. You don't care about setting up your 4th heart; you can always ruff it if you need to. You do care about winning three tricks in a row in hearts if at all possible, and starting with a heart to the ten will never achieve that.
|
Dealer North NS vul |
 A J 8 4 A J 8 4 K T 6 K T 6 T T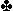 K T 6 4 2 K T 6 4 2 | |
 K T 7 2 K T 7 2 Q J Q J K 9 8 6 4 K 9 8 6 4 7 2 7 2 |
![[table marker]](../images/t.gif)
|
 Q 9 5 Q 9 5 9 8 7 2 9 8 7 2 A Q 7 3 2 A Q 7 3 2 9 9 |
 6 3 6 3 A 5 4 3 A 5 4 3 J 5 J 5 A Q J 8 5 A Q J 8 5 |
Of the 64 possible ways the missing hearts might be distributed, it's possible to avoid a heart loser against four of them: a doubleton QJ on either side, or a singleton Q or J in East.
Cashing the king and ace works against the two doubletons - a 3.24% chance. Cashing the ace first, and then finessing the ten if East drops an honour under the ace, works against three cases (winning if West has QJ or East has a singleton Q or J, but losing a trick if East has QJ) - a 4.04% chance. (It's not necessary to know the actual percentage at the table -- just to be able to figure out which line works more often.)
It's not much. But it's better than nothing. And if you bid 
Typo corrected 03 March 2010: South has the  A
A K
K
Back to HOTW index
Back to Articles index
Back to TaigaBridge home